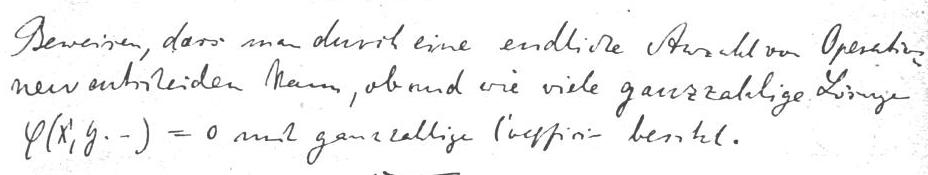
This page is under construction. Last modified
Susumu Hayashi, Humanistic Informatics, Graduate School of Letters, Kyoto university
David Hilbert's Nachlass is kept in Gottingen university library. The collection includes numerous unpublished lecture notes, letters and other documents. In the collection, there are three books of mathematical notes (Cod. Ms. Hilbert 600:1-3 in the code system of Hilbert Nachlass). They consist of short notes. Most of them are estimated to be written from 1885 to late 1910's.
These historical materials shed a new light on Hilbert's philosophy of the foundation of mathematics. After them, the primal motivation of Hilbert's foundational studies are not consistency but completeness, more accurately the conviction of "solvability of all mathematical problems" or "kein ignoramibus" proclaimed in his famous 1900 Paris lecture and repeated in his 1930 address in Königsberg. He would have had an aspiration to analyze the problem of solvability of all mathematical problems already in late 1880's or early 1890's. This idea is very likely a spin-off of his studies on invariant theory, especially Hilbert finite basis theorem, Allgemeines Endlichkeits Theorem (the general finiteness theorem) in his own words. There are notes which clearly show that his conviction of solvability was strongly influenced by Kant's philosophy. They also suggest that the conviction was his antipathy to Emil du Bois Reymond's agnosticism well-known by the phrase "Ignoramus et ignorabimus". These philosophical notes suggest that we cannot exclude even the possibility of the opposite case, i.e., his Allgemeines Endlichkeits Theorem might have been born under influences of his philosophical considerations.
A note in the book2 shows that he had already had the idea of "existence=consistency" in early 1890's, very likely before he started the series of lectures on the foundations of geometry. Another note in the book2 is an early attempt of his axiomatics. Otto Blumenthal reported that Hilbert talked about "mathematics of tables, chair, beer mugs instead of points, lines, planes" after listening to Hermann Wiener's talk on geometry at the first annual meeting of German Mathematical Society in Halle, 1891. No historical materials by Hilbert himself on this famous phrase had been known. The note include a passage "mathematics on systems of tables and dinner tables (or blackboards), etc (Tisch, Tafel, etc.)". Interestingly, his examples of "mathematics of tables and dinner tables" are not geometorical but algebraic.
Hilbert's philosophy on the foundations of mathematics must be reconsidered under the lights of these newly found historical materials. We are now studying them and we have already drawn new views of the history of the foundations of mathematics, which are rather different from the conventional ones. The research will be an international collaborations by researchers from Japan, USA, Canada and Sweden. In this web site, we publish some initial results of our researches.
Some images of Hilbert's notes and lecture notes are reproduced here under the permission of Niedersaechsische Staats- und Universitätsbibliothek Göttingen that possesses Hilbert Nachlass. We thank the library for the permission.
In this section, the original images, transcriptions, and English translations of some important notes are shown with short comments. The detailed discussions on these notes will be given in the later sections. My and Mariko Yasugi's book "The incompleteness theorem" (in Japanese) will be published by Iwanami Shoten in September 2006.The notes in this web page are mainly ones which are cited in the book. There are very many interesting notes besides these and we are planning to present such notes in this web site in future.
The estimations of time of the notes in this page are rather speculative. We have done precise philological studies of some of the notes and some rather exact estimations of the time of writing are known. However, these precise estimations could be presetned only in the form of "periods" such as "the note is written after fall of 1888 and before 10 th September 1890 ". In this web page, we did not include such a precise estimation except a few cases and give more casual speculations such as "this note seems written around 1889-1890" instead. This is because that we have not yet finished the precise philological studies for all notes, and we do not wish to be too scholastic in this web page. The precise philological researches would be published in academic papers or in books.
I thank Wilfried Sieg, Mariko Yasugi and Richard Zach for corrections and suggestions on transcriptions and tranlsations.
Hilbert's notebooks consist of three books: Cod. Ms. Hilbert 600:1-3. We will call them book1, book2, and book3. The pages of each book are numbered. We refer these page numbers below to indicate the position where the notes can be found. However, the numbering of page of the book2 is problematic. The first page of the book is numbered by zero, and the third page is numbered by 1. Thus, we will number the first two pages of book2 by 0a and 0b to avoid confusion.
From p.75 of book1, notes are mainly written by Käthe Hilbert, David Hilbert's wife. Compare the much cleaner handwriting by Käthe from the notes of 2.1.7 to to 2.2.3 with the others notes by David Hilbert himself. After David's letters to F. Klein and others, it is known that Käthe was writing David's mathematical manuscripts even before the time of their marriage. (David's and Käthe's families were quite close even before their marriage. See C. Reid's biography "Hilbert".) Occasionally, David Hilbert wrote by himself even after Käthe started to write for him. From the middle of book2, David Hilbert restart to write more and more by himself. Almost all notes of book3 are written by himself. The reason is unknown.
Hilbert's handwriting is often rather unclear and sometimes includes misspellings. Thus, transcriptions are often speculative. If we are not very sure with our guess, we indicate such as "Coeffi«ciente»" in 2.1.1 below. This means that the part surrounded by «...» is merely our guess from the context etc. and/or we are not very sure with our guess. Note that "Coefficient" is spelled "Koeffizient" in the modern standard German spelling system. However, Hilbert spells many words in different ways. We use indicate their modern and standard counterpart as "Coeffi«ciente» ‹Koeffiziente›". However, we sometime omit such annotations, when it is easy to guess the modern counterparts. We use the same notation ‹remarks or comments› to insert comments by the editors (S. Hayashi in this web page). These conventions are borrowed from Vol. 1 of Hilbert edition.
The first book is commented by David Hilbert's own handwriting "purchased in the winter 1885/86 in Leipzig". It has 133 pages.
The time of this note is estimated around 1886-7. It shows that Hilbert have conceived his famous "10th problem" already in 1880's, although it was publicized much later in his famous 23 mathematical problems of 1900.
Transcription
Beweisen, dass man durch eine endliche Anzahl von Operationen entscheiden kann, ob und wie viele ganzzahlige Lösungen Φ(x,y...)=0 mit ganzzahlige Coeffi«cienten» ‹Koeffizienten› be«sitzt».
Translation
Prove that one can decide through a finite number of operations if and how many integral solutions Φ(x,y...)=0 with integral coefficients possesses.
The notes below show that Hilbert thought about the foundations of mathematics and science based on Kant's philosophy and E. du Bois Reymond's agnosticism. The last passge of the first note in the small letters are likely added later. He is asking if the solvability of every problem could be an axiom of the human intellect. The time of these notes are estimated around 1888. I am particularly curious to know if these notes were written before he found the finite basis theorem or after it. Conclusive evidences have not been found. However, a concept of "Geschlechts" or "Geschlechtszahlen" are discussed in notes of pp. 14-15 of book1, and the concept is well-defined only when we assume the finite basis theorem. This and other indirect evidences suggest that he had already known the finite basis theorem, when he was writing the three notes below.

First note
Transcription
Ueber ein fundamentales Axiom des menschlichen Verstandes. Vielleicht dass jedes Problem lösbar ist?
Translation
About a fundamental axiom of human intellcts. Probably ‹the axiom is› that every problem is solvable?
Second note
Transcription
Begriff der ganzen Zahl beruht nothwendig ‹notwendig› auf Zeit und Raum. Begriff der Stetigkeit einfacher als als ‹two consecutive "als"s will be a mistake› der Begriff der ganzen Zahl. Kant hat Recht.
Translation
The notion of integers necessarily bases on time and space. The notion of continuity ‹is› simpler than the concept of integers. Kant is right.
Third note
Transcription
In allen anderen Wissenschaften‹,› Material, von dem man nicht weiss, wo es herkommt, von dem man nur weiss, dass es da ist. Hier reines Denken, reine Philosophie. Kant hat das als der erste gefü«hlt», aber nicht bewiesen, er hat angefangen, aber nicht zu Ende geführt.
Translation
In all other sciences, materials, of which one does not know where it comes from, and of which one only knows that it is there. Here ‹are› pure thinking, pure philosophy. Kant sensed it as the firt one, but did not prove. He began, but did not go to the end.
Two notes below are very likely to be written, when Hilbert was working on his paper "Über die Theorie der algebaischen Formen", Math. Ann. Bd. 36, pp. 473-534, 1890, since the contents of the first note of page 33 is the same asTheorem V of the page, and the formulas of the last note of the next page (p. 33) are identical to the formulas at the last part of the paper (p. 256, Gesammelte Abhandlungen, Bd 2, Chelsea, 1965).
Did Hilbert's conviction of solvability come from philosophical considerations stimulated by the finite basis theorem, or was the finding of the theorem stimulated by his philosophical considertations?

Upper note
Transcription
Vorausgesetzt, dass es keine praktischen Schwierigkeiten gäbe, ist der menschliche Verstand vermögend, alle Fragen, die er sich selbst stellt, zu lösen? Muss es möglich sein zu entscheiden über die Quadratur des Kreises, Giebt es eine wohl definirte ‹definierte›, auf die sinnlichen Dinge bezügliche Frage, welche eine Antwort haben muss und die gleichwohl sich nicht beantworten lässt.‹?› Kann man einen allgemeinen zwingenden Beweis führen, dass jede Erkenntniss «möglich» sein muss?
sinnlich: probably in Kant's sense of "Sinnlichkeit" (sensuality).
Translation
Assuming that there were no practical difficulties, is the human intellect well to solve all the questions, which it puts to itself? Must it be possible to decide on the quadrature of the circle, is there any well-defined question related to sensual things, which must have an answer and nevertheless cannot be answered? Can one show a universal and compelling proof that every cognition must be possible?
Lower note
Transcription
Wenn wir auf 2 verschiedenen Wegen widersprechende Resultate finden, muss nothwendig ein Fehler enthalten sein. Was würden wir denken müssen, wenn kein Fehler sich findet? Wir werden diesen Fall als unrealisirbar betrachten. Müssen wir in gleichen Sinne auch den Fall als unrealisirbar betrachten, dass sich etwas nicht beweisen (entscheiden durch endliche Zahl von Schlussen lässt)?
Translation
When we find contradictory results in two different ways, a mistake must necessarily be contained. What would we have to think, if no mistake is found?
We will consider this case impossible. Do we have to consider, in the same sense, the case impossible where something is never proved (decided through a finite number of deductions)?
Pages 32 and 33


The time of the note is estimated in 1888-1889. This would be one of the most interesting notes in the three notebooks. Hilbert clearly claimed that every mathematical problem is solvable (lösbar) by finite number of operations. A problem if ten consecutive 7 appear in the constant π, which is almost the same as Brouwer's "counterexample" of the law of excluded middle, is mentioned.

Transcription
Jedes Mathem. Problem kann auf folgende Frage zurückgeführt werden: Gegeben ist ein Gesetz, vermöge dessen zu jeder vorlegten ‹vorgelegten› nur aus Null und 1 bestehenden nicht abbrechenden Reihe
(α) 0 0 1 1 0 0 ...
eine bestimmte andere solche Reihe (durch Rechenoperationen: Grösste Ganze suchen, Teilersuche etc. nicht Würfeln...)
(β) 1 0 0 1 1 1....
construiert ‹konstruiert› werden kann. Man soll durch eine endliche Zahl von Operationen entscheiden ob in irgendeiner Reihe (β) eine 0 vorkommt oder ob alle Reihen (β) nur ‹aus› Einsen bestehen?
Ich behaupte: Jede solche Entscheidung ist durch eine endliche Zahl von Operationen (Rechenoperationen) möglich«,» d.h. es giebt kein Gesetz, bei welchem die Entscheidung nicht durch eine endliche Zahl von Operationen möglich wäre. D.h. jedes math. Problem ist lösbar. Alles dem menschlichen Verstande erreichbare (durch reines Denken ohne Materie) ist auch aufzulösen. Es giebt nur ein Problem. (z.B. Quadratur des Kreises, hat π=3,14... 10 aufeinander folgende 7en, etc.) Von der Annahme der Möglichkeit geht man von vorne herein ‹vornherein› aus.
Translation
Every mathematical problem can be reduced to the following question: A rule is given, by which to
every given non-breaking off sequence consisting only of zero and 1
(α) 0 0 1 1 0 0...
a certain other such sequence (by computational operations: «Grösste Ganze Suchen», etc. not throwing dices...)
(β) 1 0 0 1 1 1....
can be constructed. One is to decide by a finite number of operations whether if zero occurs in some sequence (β) or all sequences (β) consist of only ones.
I claim: every such decision is possible through a finite number of operations (computational operations), i.e. there is no rule, with which the decision would not be possible by a finite number of operations, i.e. every math. problem is solvable. All that the human intellect can reach (by pure thinking without matters) is also to be dissolved. There is only one problem (e.g. quadrature of the circle, π=3,14 has... 10 successive 7's etc.) One proceeds at the outset from the assumption of the possibility.

Transcription
Die Mathematik ist die einzige Wissenschaft, bei welcher wir nicht@ignorabimus‹, › sondern im Gegenteil im «weiteresten» ‹weitesten› Umfang in Bezug auf@alle und noch so schwierigen Probleme sagen müssen: noscemus.
Translation
Mathematics is the only science where we are not "ignorabimus", rather, on the contrary, with the furthest extent, concerning to all and even very difficult problems, we must say "noscemus".
ignorabimus: We shall not know. (Latin)
noscemus: We shall know. (Latin)
Hilbert's student Otto Blumenthal reported in his Lebensgeschichte for Hilbert that Hilbert said "Man muß jederzeit an Stelle von 'Punkte, Geraden, Ebenen' 'Tische, Stühle, Bierseidel' sagen können" ("One must always be able to say 'tables, chairs, beer mugs' instead of 'points, line, when he was discussing with other mathematicians in a waiting room of Berlin station. They would be on the way back to Königsberg from Halle, where they attended the first annual meeting of German Mathematical Society 1891. In the meeting, Hermann Wiener, a mathematician at Halle university talked about geometry and it is said that Wiener's talk stimulated Hilbert's axiomatics. However, any historical materials by Hilbert himself on this famous story had not been known. In the following note, Hilbert talked about "mathematics on systems of tables and dinner tables (or blackboards), etc (Tisch, Tafel, etc.)". Interestingly, his examples of "mathematics of tables and dinner tables" are not geometrical but algebraic.


Transcription
Mehrere Dinge zusammen in einem Begriff gefasst, geben ein System‹,› z.B. Tisch, Tafel, etc... In der Mathematik betrachten wir Systeme von Zahlen oder von Funktionen. Dieselben brauchen nicht in abzählbare Menge zu sein. Das System ist vielmehr gegeben und bekannt, wenn man ein Gesetz kennt, vermoege dessen von einer vorgelegten Zahl oder Funktion entschieden werden kann, ob dieselbe zu dem System gehoert oder nicht. Beispiele. Die Systeme koennen Eigenschaften haben z.B. invariante Operationen z.B. Alle Funktionen gehören «w»ieder zum System, die man erhaelt, wenn man a) + (z.B. ositiven Lösungen von linearen diophantischen Gl.‹Gleichungen›) b) +- c) + und * (z.B. System aller definiter Formen) d) +- * (Integritaetsbereich) e) +- * : (Rationalitaetsbereich). Endliche - unendliche Basis -- Systeme von Systemen.
Translation
Serveral things combined into a concept, gives a system, for example: table, blackboard (or dinner table) etc. In mathematics, we regard systems of numbers and functions. They need not to be in countable sets. The system is rather given and known, if a rule is known, with which a given number or function can be decided, whether it belongs to the system or not. For Example. The system can have the properties, e.g. invariant operations, e.g., ‹values of› all functions, which one gets, belong again to the system, when one ‹applies› a) + (e.g. positive solutions of diophantos equations) b) +- c) + und * (eg. Systems of all efinite forms) d) +-* (Integral domain) e) +-*: (Rational domain). Fine- Infinite bases _ System of systems.
Rational domain: an old name of "field"


Transcription
Zu Gordan sagen: Wenn Sie kommen, so weiss ich entweder, es ist Prof. Gordan, oder es ist nicht Prof. Gordan. Sie aber würden sagen: oder drittens. Die kommende Person ist Keins von Beiden.
Von meinen Invariantentheorischen Sätzen folgendes sagen:
1) Die Symbolische Rechnung und *erei ‹Rechnerei› in der Invariantentheorie stets an meinen Sätzen in de«n»selben Verhältniss ‹demselben Verhältnis› wie die Jakobische Rechnerei in der Theorie der elliptischen Funtionen zu der nachfolg. ‹nachfolgenden› Theorie von Weierstrass oder von Riemann‹,› wo durch ‹this word is not necessary?› die Funktion durch die Nullstelle bestimmt wird.
2) Die Symbolik hat‹,› so kann man mit «ein»iger Übertreibung sagen‹,› der Invariantentheorie geschadet, da meine Sätze sonst vielleicht «schon» f«rü»her entdeckt wären, wie die Erfindung des Luftballons dem Fortschritt in den Bestrebungen eine Flugmaschine zu erfinden geschadet hat.
Translation
Say to Gordan: If you come, then I know either, it is Professor Gordan, or it is not Professor Gordan. You would however say: or thirdly. The coming person is none of both.
On my Invarianten theory theorems, ‹I› say followings:
1) the symbolic calculation and repeated calculations in the invariant theory ‹are› always in my theorems in the same proportion that Jacobi's calculation in the theory of the elliptical function ‹are› in the succeeding theory due to Weierstrass and Riemann, where the funtion is determined by root‹s›.
2) one can say with some exaggeration that the symbolism has damaged the invariant theory, as the invention of the balloon damaged the progress of the efforts of invention of airplanes, since my theorems would be discovered earlier instead.
The second book is commented as " Professor David Hilbert Augustastr. 4, 12 November 1892" likely by Käthe Hilbert's hand.
This note show that Hilbert's interests on computations in mathematics. He thought even an idea resembling the modern concept of computational complexity.


Transcription
Stelle auf eine Theorie des Rechnens d.h. ergrüde worin die Vortheile ‹Vorteile› bekannter arithmetischer Formeln und Sätze (Summation arithmetischer Reihen, Congruenz‹Kongruenz›-formeln, Stirlingsche Formel, vergl. Schlömilch Compendium Bd 2 S.264.) zum Ausrechnen bestimmter Aufgaben beruhen und wie man diese Vortheile rechnerisch abschätzen kann. Vielleicht indem man gewisse elementare Rechnungsoperationen (addiren ‹addieren›, multipliciren ‹multiplizieren›, potenziren ‹potenzieren›) als Einheiten einfüuhrt und die Zahl der anzuwendenden Einheiten auf verschiednen Wegen, die man beim Rechnen einschlägt, bestimmt. Wie viel Rechnungeinheiten z.B. sind nötig, um die so und so vielte Stelle der Decimalbruch-Entwicklung von π zu bestimmen? Wie berechnet man n! am schnellsten? Vielleicht die ersten Ziffern aus Stirlings Formel, und die letzen durch Congruenz‹Kongruenz›-Rechnung.
Translation
Set up a theory of calculations i.e. find out on what the advantages of well-known arithmetic formulas and theorems (summation of arithmetic series, congruence-formulas, Stirling's formula, cf. Schlömilch's compendium vol.2 p.264) to calculate certain tasks are based and how one can estimate those advanteges by way of calculation. Perhaps ‹that would be done› by introducing certain elementary calculation-operations (add, multiply, power) as units and determining the number of units to be used in different ways, by which one takes in the calculation. How many calculation-units, for example, are necessary in order to decide an arbitrary place of decimal-development of π? How does one calculate n! in the fastest way? Perhaps the first numbers by Stirling's formula, and the last ‹numbers› through congruence-calculation.
This note is estimated to be written around 1893. Hilbert clearly defined the existence by consistency of its "Merkmale (Axiome)". This is puzzling, since he cited Cantor's idea of "consistent sets exist", when he described the same idea in 1900. Hilbert and Cantor had discussions on the subject through letters in the late 1890's. This note seems older by 3 or 4 years than them. What are the relationships of the note and the discussion?

Transcription
Existiren ‹Existieren› heisst die den Begriff definirenden ‹definierenden› Merkmale (Axiome) widersprechen sich nicht, d. h. es ist nicht möglich aus allen mit Ausnahme eines durch rein logische Schlüsse einen Satz zu beweisen, welcher dem letzten Axiom widerspricht. Man braucht jedoch, zumal im gewöhnlichen Leben existieren auch gleich für die Beschreibung der Dinge besonders forderlich sein, z. B. Gott existirt ‹existiert› nicht, d.h. das Axiom Gott ist, ist zur Beschreibung vieler Erscheinungen überflüssig
Translation
To exist means that the conditions defining the concept do not contradict to themselves, i.e. from all ‹axioms› with an exception ‹an exceptional axiom›, it is not possible to derive a proposition, which contradicts to the last ‹exceptional› axiom. One needs however, particularly in ordinary life "exist" is also equally for the description of the things particularly useful, e.g. God does not exist, i.e. the axiom "God exists", is superfluous to the description in many cases.

Transcription
Alles was Gegenstand des Denkens ist, ist daher Gegenstand der Mathematik. Die Mathematik ist nicht die Kunst des Rechnens, sondern die Kunst des Nichtrechnens.
Translation
Anything, which is object of thinking, is therefore object of mathematics. The mathematics is not the art of computation, but the art of non-computation.
This note, which is estimated to be written in late 1910's, show that his interest in constructivization of existence theorems in mathematics. In the 1917 paper "Axiomatisches Denken", Hilbert discussed the problem by citing his non-constructive solutions of Gordan's theorem by the finite basis theorem and its "constructivization" by Nullstellensatz, and an example from y Rohn's research in algebraic geometry in length. In the paper, the word "Entscheidung" was used as "determination of values". It was H. Behmann, a student of Hilbert, to use the word "Entscheidung" in the modern sense of Entscheidungsproblem. It was, however, in 1920's. (Cf. P. Mancosu, "Between Russell and Hilbert: Behmann On the Foundations of Mathematics", Bulletin of Symbolic Logic, Vol. 5, 1999.) The word second to the last is very unclear from the facsimile that we possess.
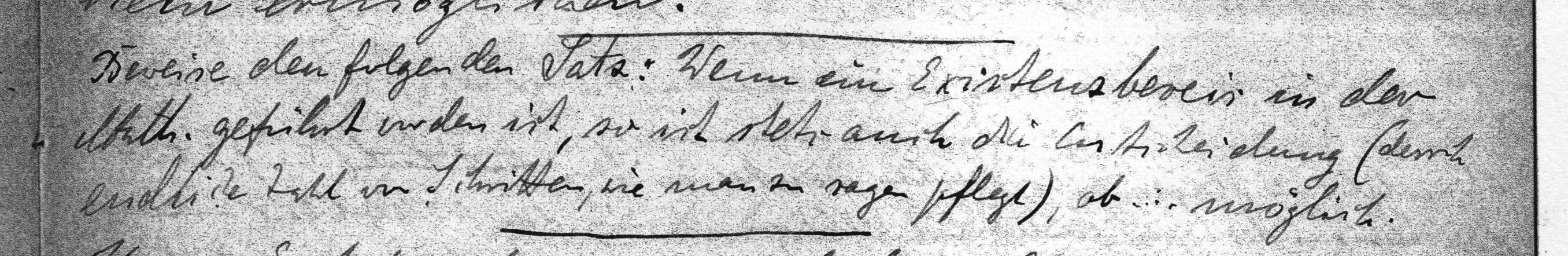
Transcription
Beweise den folgenden Satz: Wenn ein Existenzbeweis in der Math. geführt worden ist, so ist stets auch die Entscheidung (durch endliche Zahl von Schritten wie man zu sagen pflegt), ob «...» ‹it can be a spot› möglich.
Translation
Prove the following theorem: if an existence theorem is once derived in the mathematics, so is always also the determination ‹Entscheidung›(through a finitie number of steps as they are used to say), or possible.
To be written....
4. Thoughts on computation
Computation theory note, Non-computational mathematics, Constructivization note